IEC 60287 Current Capacity of Cables - Rated Current
This note looks at the formulae used to calculate the rated current capacity of a cable in line with IEC 60287 "Calculation of the continuous current rating of cables (100% load factor)". Before you continue reading this note, if you have not done so already we would suggest first reading our IEC 60287 introduction note:
IEC 60287 Current Capacity of Cables - An Introduction
In the previous note we looked at the approach taken by the standard to the sizing of cables and illustrated this with an example. We then looked at one method of applying the standard and identified resources enabling the calculation of all the various parameters involved. In the note we are going to put everything together and reveal the necessary equations for actually calculating the cable maximum current rating.
The image illustrates the thermal model for a cable.
_thumb.png)
Heat is generated within the cable by various mechanisms - conductor I2R loss, dielectric loss, sheath loss, armour loss and direct solar radiation. Some or all of this heat is dissipated through the cable insulation, bedding, serving and into the surrounding medium. The rate of heat flow is related to the temperature difference across the cable and affected by the ambient temperature, temperature rises due to other cables and any critical temperature rise of the soil above ambient.
In thermal equilibrium, when all these factors have balanced and the temperature of the conductor is the maximum allowable for the insulation; we have the maximum rated current for the cable. It can probably be appreciated by now, that in typical real life situations, this can be quite a complicated calculation.
Note: rated current capacity found by the method assumes that the cable is fully loaded for 100% of it's operation time. For cables which have varying or cyclic loads, the current rating could possibly be increased.
Tip: the thermal model is worth remembering as enables us to intuitively understand how a cable is likely to behave in conditions which are not normally encountered. For example, if a cable is run along a refrigerated gas pipe, we can hazard that this will reduce the ΔΘ and hence the cable will be able to carry more current.
Change in temperature across a material is equal to the heat input multiplied by the thermal resistance of the material. In terms of the thermal model and for a simple a.c. cable, the heat balance equation is given by (a list of symbols is given at the end of the note):
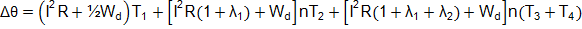
Within the standard this above is used to derive the equations for current rating. It is simplified for d.c. cables by eliminating any a.c. only effects and modified for partial drying of soil and solar radiation where appropriate.
Rated Current of Cables
The standard gives the following equations for the calculation of the cable current rating (for all alternating current voltages and direct current up to 5 kV):
Buried cables where drying out of the soil does not occur or cables in air
AC cables

DC cables
_thumb.png)
Buried cables where partial drying-out of the soil occurs
AC cables
DC cables
Buried cables where drying-out of the soil is to be avoided
AC cables
DC cables
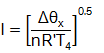
Cables directly exposed to solar radiation
AC cables
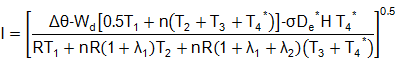
DC cables
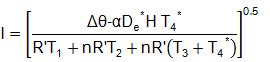
Note: when calculating a cable where some drying of the soil may occur, it is also necessary to perform the calculation for no drying out of soil and take the worse case (lower) rating.
The calculation of each element needs some explanation and these have been split across several notes (with each note dealing with one topic). For details, please refer to the first note in this series, which lists the other related notes.
List of Symbols
n - number of load carrying conductors
v - ratio of thermal resistivity of dry and moist soils
I - rated conductor current, A
R - a.c. resistance of the conductor per unit length, Ω/m
R' - d.c. resistance of the conductor per unit length, Ω/m
T1 - thermal resistance per core between conductor and sheath, K.m/W
T2 - thermal resistance between sheath and armour, K.m/W
T3 - thermal resistance of external serving, K.m/W
T4 - thermal resistance of surrounding medium, K.m/W
T4* - external thermal resistance (free air) adjusted for solar radiation, K.m/W
De* - cable diameter over insulation, m
H - intensity of solar radiation, W/m2
Wd - dielectric loss per units length, W/m
λ1 - ratio of losses in metal sheath to total losses in all conductors
λ2 - ratio of losses in armouring to total losses in all conductors
σ - absorption coefficient of solar radiation for cable surface
Θ - maximum conductor operating temperature, °C
Θa - ambient temperature, °C
ΔΘ - temperature difference (Θ-Θa), K
ΔΘx - critical temperature of soil, °C